Generator of finite cyclic group

In algebra, a cyclic group or monogenous group is a group that is generated by a single element.^[1] That is, it consists of a set of elements with a single invertible associative operation, and it contains an element g such that every other element of the group may be obtained by repeatedly applying the group operation or its inverse to g. Each element can be written as a power of g in multiplicative notation, or as a multiple of g in additive notation. This element g is called agenerator of the group.^[1]

Every infinite cyclic group is isomorphic to the additive group of Z, the integers. Every finite cyclic group of order n is isomorphic to the additive group of Z/nZ, the integers modulo n. Every cyclic group is an abelian group (meaning that its group operation is commutative), and every finitely generated abelian group is a direct product of cyclic groups

The six 6th complex roots of unity form a cyclic group under multiplication. zis a primitive element, but z²is not, because the odd powers of z are not a power of z².

Infinite cyclic groups

p1, (*∞∞)	p11g, (22∞)
Two frieze groups are isomorphic to Z. With one generator, p1 has translations and p11g has glide reflections.

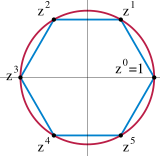
A group G is called cyclic if there exists an element g in G such thatG = ⟨g⟩ = { gⁿ | n is an integer }. Since any group generated by an element in a group is a subgroup of that group, showing that the only subgroup of a group G that contains g is Gitself suffices to show that G is cyclic.

For example, if G = { g⁰, g¹, g², g³, g⁴, g⁵ } is a group of order 6, then g⁶ = g⁰, and G is cyclic. In fact, G is essentially the same as (that is, isomorphic to) the set { 0, 1, 2, 3, 4, 5 }with addition modulo 6. For example, 1 + 2 ≡ 3 (mod 6) corresponds to g¹ · g² = g³, and2 + 5 ≡ 1 (mod 6) corresponds to g² · g⁵ = g⁷ = g¹, and so on. One can use the isomorphism χ defined by χ(gⁱ) = i.

The name "cyclic" may be misleading:^[2] it is possible to generate infinitely many elements and not form any literal cycles; that is, every gⁿ is distinct. (It can be thought of as having one infinitely long cycle.) A group generated in this way (for example, the first frieze group, p1) is called an infinite cyclic group, and is isomorphic to the additive group of theintegers, (Z, +).

Nicolas Bourbaki, who was the French mathematicians group, called a cyclic group as monogenous group,^{[note 1]} and defined a finite monogenous group as a cyclic group,^{[note 1]} then, Bourbaki avoided the term infinite cyclic group